Understanding TWR vs IRR Return Calculation Methodologies In Performance Reporting Software

Calculating A Proper Performance Rate Of Return

Conceptually, a rate of return is quite simple. It starts with the answer to a simple question “How much money have I made on my investment?”  And actually, that number is pretty easy to determine.

But the next question isn’t so easy, which is “What is that amount as a percentage of my investment?” That number is significant for two reasons.  First, it allows you to evaluate how a portfolio has done historically, and compare it with benchmarks (i.e., had a similar investment been made into the benchmark instead). Secondly, it allows you to estimate future earnings, and therefore model retirement strategies.

The problem with calculating that percentage often comes down to determining the investment or basis for the performance, which is influenced by cash flows. And oftentimes, there can be a tradeoff between the ease of understanding a “simple” rate of return, versus a portfolio’s “true” performance… especially when there are large deposits into (or withdrawals from) the portfolio along the way.

 The Impact Of Cash Flows On Investment Performance

The root of all the differences in performance calculations is how they treat cash flows into and out from a portfolio. If a client were to simply give an advisor some money to invest, and never add to or take away from that initial investment, then performance calculations would be very straightforward. In fact, all methods of determining performance will give you the same result if there are no external cash flows in/out of a portfolio.

However, that is rarely the case in practice, and each method of computing investment performance – a basic return calculation, a time-weighted return, or a dollar-weighted return – handles those cash flows differently.  Each one attempts to account for those flows in different ways, and will provide a performance number that reflects a different point of view.

For example, a time-weighted rate of return is designed to eliminate the effects of cash flows, and just scores the allocations or strategy, whereas a dollar-weighted (or money-weighted) rate of return embraces and includes the cash flows (and provides a rate of return that reflects the timing and sizes of those cash flows).

Performance Calculation Objectives

Ultimately, the different methods of computing performance exist in order to measure different things.

A basic rate of return (total amount of growth divided by the initial account balance) provides a simple, but not always accurate, measurement of investment growth (due to the impact of cash flows along the way).

A time-weighted return, by contrast, is designed to measure the results of your overall investment strategy, or model, or allocation, as it is applied to a portfolio. In other words, it’s intended to strip out any impact of cash flows that the client may have made to/from the portfolio, so you can calculate a “pure” investment performance directly attributable to your actual investment decisions as the manager of the portfolio holding the various underlying securities.

A dollar-weighted rate of return provides a more complicated, albeit also more accurate, measurement of actual investment growth and results relative to the dollars that were invested both upfront and along the way. Or viewed another way, while a time-weighted return is a reflection of the returns of the investment strategy, a dollar-weighted return represents the investor’s personal return from implementing the strategy (including the impact of any additions or withdrawals they made along the way).

Thus, the question of “which is the right methodology for calculating performance results?” depends on whether you are looking for a growth amount you can plug into future financial planning projections, are trying to justify or highlight the value and results of your investment strategy, or are trying to give a client an understanding of how his or her own investments are doing (given what the client added or subtracted in cash flows along the way? The answer to this question can guide you to the proper performance calculation method.

Methods of Computing Investment Performance: Basic, TWR, and DWR/IRR

As noted earlier, there are three main methods for computing investment performance results:

Basic (or Simple) rate of return: This is easy to calculate, and easy to understand. It simply divides the change in value over the time period, by the starting value at the beginning of that time period. There are a couple of standard ways it can handle flows (e.g., subtracting out any intervening cash flows, or just calculating the amount of “growth” in the form of interest, dividends, and capital gains, and dividing by the starting value). However, the return can be skewed when there are a lot of inflows and outflows along the way.

Time-Weighted rate of return (TWR): There are several ways of computing TWR, but the “Modified Dietz method” is the most common. In a TWR calculation, you compute returns for smaller discreet time frames (like one month at a time) and then geometrically link the returns of those time periods together to calculate the return for the entire time horizon. By breaking up performance into smaller units, this method will minimize or eliminate the effect of cash flows.

Dollar (or Money) Weighted rate of return (DWR): Also called an Internal Rate of Return (IRR), this method will account for any inflows or outflows as they happen, and compute an overall rate of return over time by weighting each time interval by the amount of cash invested at any given time (effectively taking into account each cash flow). It is by far the most complicated and confusing calculation, but it will give you a more meaningful measurement of the investor’s actual returns in the portfolio. 

Basic Rate of Return

The basic rate of return provides you and your client a simple rate of return that is easy to compute and easy to understand. In a lot of cases, it is an adequate representation of the performance of a portfolio (especially if there were minimal inflows or outflows along the way).

The basic rate of return takes the gain for the portfolio and divides by the (original) investment amount.  If there are no flows to a portfolio, then you simply take the Ending Value (EV) and subtract the Beginning Value (BV) to get the gain (or loss), and then divide by that starting value.

Basic Return = (EV – BV) / BV

If there are cash flows, you have to decide what to do with them. The real question is “did those flows ‘earn’ part of the gains or not?”. One method is to assume the Cash Flows (CF) did take part in performance, which basically treats the flows as though they happened at the beginning of the performance period, and the formula is modified to look like this:

Basic Return = (EV – BV - CF) / (BV + CF)

Thus, the Cash Flows are removed out of the equation when calculating the gain (because contributions aren’t a “return”, they’re simply a contribution), and are included as part of the starting balance. Which means the net growth is assumed to have occurred on both the starting balance, and the contributions themselves. (If the Cash Flows were withdrawals, the same adjustments would occur, simply in the opposite direction as outflows.)

Another method assumes that the flows did not take part in performance, as though they occurred at the very end of the performance period. The formula is modified to look like this:

Basic Return = (EV – BV - CF) / (BV)

Of course, the reality is that cash flows in/out of a portfolio rarely actually occur precisely on the first or last day of the time period, which means assuming the cash flows had occurred in time to cover all (or none) of the time period will not be entirely accurate.

There are other Basic Return methods that assume the cash flows happened halfway through the performance period, or attempt to weight the flows, but ultimately these quickly add complexity to the calculation, and at that point, you would probably be better off just using an IRR dollar-weighted performance calculation instead (as discussed below).

The Basic Return calculation remains viable – even when there are interim cash flows – when the amount of the cash flows that move in/out of the portfolio are modest relative to the total size of the portfolio (such that, mathematically, the returns aren’t distorted very much). Accordingly, the real issue with a Basic return is when there are large withdrawals from (or contributions to) a portfolio.

For example, consider a portfolio that starts with $100,000, and then over the year goes up to $120,000, but the investor sells off $90,000 toward the end of the year to use the money and finishes with a balance of just $30,000. The portfolio “made” $20,000, but the starting investment, after adjusting for the withdrawal, would be $10,000, which would make it look like the portfolio experienced 200% growth!

Of course, if it was assumed in the Basic Return calculation that the cash flows all occurred at the end of the year, then the growth would have been $20,000 of gains on a $100,000 starting balance (not adjusted for the cash flows up front), which would yield a more ‘intuitive’ 20% return on the portfolio. Except if the withdrawal had actually occurred mid-year, neither may be an accurate reflection of the growth that happened throughout the year.

To say the least, when looking at portfolios with large withdrawals (or contributions), the basic rate of return can exaggerate gains or losses.

Time-Weighted Rate of Return (TWR)

A time-weighted return attempts to minimize or altogether remove the effects of interim cash flows during the performance measurement period.

The concept of a TWR is that it measures the performance of the holdings in a portfolio without the influence of inflows or outflows, so that the “pure” performance of the underlying manager can be compared to benchmarks or other portfolios that are allocated in a similar fashion. By doing this, a TWR is not focusing on the true performance outcomes for this portfolio and for this client in particular, but rather it is more focusing on the performance of the underlying strategy and allocation behind the portfolio.

To accomplish this, a TWR breaks up the performance period into multiple time frames (typically a month at a time), computes the performance for each month separately, and then finally links them back together for overall performance for the whole time period. By doing so, it makes each month “equally important” for performance, regardless of the value at the end of each month.

Thus, in a case where a person is contributing $1,000 per month, the performance for the first month, with only $1,000 in it, is equally as important, or “weighed” the same, as the performance in month 12 which has $12,000 in contributions, or in month 200 that has $200,000 in cumulative contributions. Which, notably, means that a 2% return in the first period (earning $20 on a $1,000 balance) is treated as the same 2% return as in the last period (where a 2% return would generate $4,000 on a balance of $200,000)… even though the actual returns were $20 and $4,000, respectively.

There are two steps to computing a TWR:

• Compute the performance for each individual month (separately). Even at the monthly level, there is still a question of how to handle intra-month cash flows. If cash flows regularly occur at the beginning or end of the month, a Basic Return calculation may be sufficient (and if cash flows are modest in any particular month, may be “close enough”). With more cash flows, an IRR calculation (discussed below) for each month may be more appealing.

Most commonly, investment performance reporting software will use what is called the Modified Dietz formula to calculate the monthly returns, which is similar to the Basic Return formula, but weights the amount of intra-month Cash Flows that are treated as part of the Beginning Value by the number of days that the cash flow was in/out of the account. For instance, a $100,000 account that gets a $3,000 contribution on the 29^th day out of 30 is treated as though it had a starting balance of $100,000 + 1/30^th of $3,000 = $100,100.

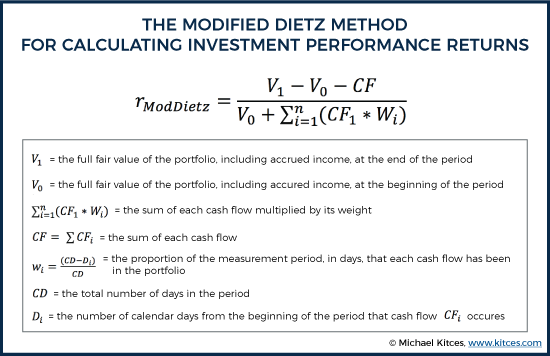
There is also a Simple Dietz method which simply assumes cash flows occurred at the midpoint of each time period.

• Link each month together. To get the overall performance based on individual monthly performance, the second step is to combine the monthly performance values into a single compounded return, which is typically called “linking”. To do this, add 1 to each monthly performance value (e.g., a 1% return becomes 1.01, an 8% return becomes 1.08, etc.), then multiply them all together, then subtract 1 from the result (to convert it back to a percentage return):
  • TWR = (1 + m1) x (1 + m2) x …x (1 + mN) - 1

In the logical extreme, it’s also possible to calculate a “true” Time-Weighted Return, by breaking up the performance into separate segments whenever there is any cash flow (rather than just on a monthly basis), and then link those segments together. Historically, such calculations were too time-intensive to be practical or feasible (and require more information to capture not just monthly balances and cash flows but the daily balance of the account over the entire time horizon), although with software making performance reporting calculations easier, the methodology may become more common in the future.

The bottom line, though, is that a TWR calculation attempts to minimize the effects of cash flows, in order to standardize the performance calculation for a portfolio and get at the underlying performance of the strategy. This allows a true apples-to-apples comparison of performance of the strategy itself relative to benchmarks and other similarly allocated portfolios, and is required for GIPS compliance (see below).

However, the TWR approach does not reflect the effects of the investor’s market timing contributions to or withdrawals from a portfolio, and as such, it is not necessarily the best indicator of the individual investor’s true returns on their actual invested dollars.

Dollar-Weighted (or Money-Weighted) Rate of Return (IRR)

A dollar-weighted rate of return, or IRR, is a complicated formula that determines an internal rate of return that would “explain” your gains or losses on a portfolio. It really isn’t something that a person could typically calculate by hand, unless there are no cash flows (and at that point, the results would be the same as a TWR or Basic Return calculation anyway).

When there are cash flows, the DWR (or IRR) methodology segments the performance period into time frames for each cash flow in/out of the portfolio, weights each time frame by the amount of money in the portfolio over that time period, and then determines the single rate of return across those variously-dollar-weighted time periods that would get you to the actual ending value of the portfolio.

The actual calculation of IRR generally involves an initial “guess” to estimate the return, and then an iterative approach to finding the exact rate (by incrementing the initial guess up or down and applying it to the dollar-weighted time frames until the calculation arrives at the actual ending value of the portfolio). Financial advisors may be familiar with this approach from the “Solve” function in Excel and other spreadsheet software.

Unfortunately, the iterative nature of the IRR calculation means that if a client were to want to see the exact computation, you would be hard pressed to provide that for him/her. The best option would be to create an Excel Spreadsheet, and enter in a starting value, all flows and their dates, an ending value, and then use Excel’s IRR function to calculate the performance result (and be able to show what each of the underlying cash flows were, and the starting and final balances, to arrive at that return result). Or simply apply the already-calculated IRR return to the various time periods, weighted by their dollar-adjusted cash flows, to “show” that the IRR return really does provide the appropriate final result. (To say the least, it is not ideal as a method to easily validate the calculation! Though fortunately many spreadsheet and performance reporting solutions are available to help!)

Nonetheless, if the client isn’t interested in how you mechanically computed the number, but simply wants the best indicator of their actual investor return performance, which includes market timing and cash flows, it is the best method for computing performance.

Method Of Performance Reporting Case Study

Consider the following scenario that we will use to highlight the different computation methods, using the fictitious ABC company.

• On January 1^st, you purchase 10,000 shares of ABC at $10 per share
  • Total investment of $100,000
  • Portfolio value is $100,000 (10,000 @ $10)
• ABC grows to $20 per share by Jan 31
  • Portfolio value is $200,000 (10,000 @ $20)
  • You have made $100,000 in January
• On February 1^st, you purchase 10,000 shares at $20 per share
  • Cash flow of $200,000, total investment of $300,000
  • Portfolio value is $400,000 (20,000 @ $20)
• On February 28^th, ABC shares drop to $14
  • Portfolio value is $280,000 (20,000 @$14)
  • You have lost $120,000 in February.
  • You have lost $20,000 lifetime.

In this example, we purposely put the flows at the beginning of the month, so the monthly calculations would be a bit easier. In this scenario:

January: All 3 methods will yield the same thing.  We started with $100,000, we ended with $200,000, and since there were no intervening cash flows we are up $100,000 on an investment of $100,000 and it’s all pure investment return, so we have a rate of return of 100%.

February: Since we put the flow at the beginning, all three methods will show the same result for the month of February.  We started with $400,000 (since the contribution happened precisely at the beginning of the month), and ended with $280,000, which means we lost $120,000 and have a rate of return of -30%.

Year to Date: The monthly returns are easy to follow, but now let’s look at YTD:

• Basic Rate of Return: The investor cumulatively invested $300,000 (which is $100,000 initially plus $200,000 in the second month), and lost $20,000 (which is $280,000 final value, minus $100,000 starting value, and $200,000 of cash flows), so the basic rate of return is -6.67%.
• Time-Weighted Return: January was up 100%, while February was down 30%, which when time-linked gives us a rate of return of +40%.
• IRR (Dollar-Weighted Return): The IRR calculation for this scenario is rate of return of -10.22%, which reflects the unfortunate fact that while the gain was 100% in January and the loss was “just” 30% in February, the loss receives a much higher weighting because the investor added dollars to the portfolio just before the loss and had far more invested (therefore, a higher dollar weighting) on the way down. (Notably, this IRR calculation is not the same as would be calculated in Excel, as the Excel IRR calculation assumes equal time periods and is annualized, whereas this example includes time periods with a slightly different number of days, and was only for 2 months and not a full year.)

ABC Company: Also worth noting is what the underlying company holding itself did. The ABC company started the year at 10 as now at 14, which means it is up 40% for the year.  NOT coincidentally, this is the same return shown by the TWR (which is meant to represent the returns of the underlying portfolio, regardless of the investor’s cash flows in or out).

This scenario highlights some of the differences in the methods of computing performance. The IRR shows a larger loss than the basic rate of return.  This is because the IRR accounts for the fact that the additional $200,000 contribution in February was participated in the market decline in February but not the gain in January (thus increasing the weighting of the February loss), whereas the basic does not make that distinction that part of the cash was only invested for the loss but not the gain. The IRR of -10.22% is a better indicator of the true performance than the basic, which is -6.67%.

At the same time, you are probably tempted to look at the TWR and assume something is drastically wrong.  How can their performance be a positive 40% on the year, when the portfolio is clearly down (especially with the big contribution in February right before the portfolio declined)?  But that is what a TWR is intended to measure: it eliminates the effects of cash flows, and looks at the underlying holdings.  There was only 1 holding, and it actually was up 40% for the time period (from a starting price of $10/share to the final price of $14/share), and so the TWR reflects that result (the performance of the underyling holding).

GIPS Compliant Performance Reporting

GIPS is an organization that has created voluntary standards and guidance for the financial industry. Because GIPS compliance is a voluntary process that an advisory firm many undertake with a third party, the firm’s policies and procedures are what is evaluated to determine “GIPS compliance”, and not necessarily the performance reporting software being used. Instead, performance reporting software is expected to conform to GIPS standards in order to produce GIPS-compliant performance reports.

Because GIPS is intended to be used to provide standardized performance reporting results to evaluate and compare managers, it requires a time-weighted calculation method, and has specific requirements about how to calculate those time-weighted results. The Modified Dietz method adjusts for cash flows daily (by calculating a daily-weighted contribution of the cash flow), and so that method fits the GIPS requirements as specified in the current GIPS rules:

The GIPS standards require a time-weighted rate of return because it removes the effects of external cash flows, which are generally client-driven. Therefore, a time-weighted rate of return best reflects the firm’s ability to manage the portfolios according to a specified mandate, objective, or strategy, and is the basis for the comparability of composite returns among firms on a global basis.

Time-weighted total return calculation methods that adjust for external cash flows in the portfolio using the mid-point or mid-period methods, such as the Original Dietz method, are acceptable for calculation periods prior to 1 January 2005. Because the philosophy of the GIPS standards is to present performance that is as accurate as practically possible, the GIPS standards have transitioned to more precise calculation methodologies. Therefore, firms must use time-weighted total return calculations that adjust for daily-weighted external cash flows for calculation periods beginning on or after 1 January 2005 at the latest; firms may use this methodology for calculation periods prior to this date.

For periods beginning on or after 1 January 2010, firms must value portfolios on the date of all large cash flows, in addition to calendar month-end or the last business day of the month; firms may use this methodology for calculation periods prior to this date. Performance is then calculated for sub-periods. The sub-period returns are then geometrically linked to calculate the total period total return … Once a calculation methodology is selected for a portfolio, it must be used consistently until the firm transitions to a more accurate methodology.

Conclusion

Deciding how to compute performance for your clients’ portfolios is not always an easy task. Questions such as…

• “Are you looking for a simple number that your client understands?”
• “Are you trying to highlight your models and strategies?
• “Are you trying to be GIPS compliant?”
• “Are you trying to plan for the future based on past performance”

…all play a role in the decision-making process.

The first step is to understand what the different performance calculation methods are trying to measure, and how they calculate the rate of return.  Armed with that knowledge, you can choose a method that best fits what you are trying to communicate with your clients.

In practice, advisory firms will typically want a means to calculate both a time-weighted return (particularly if they are advertising the actual results of their investment strategies, and/or want to voluntarily show a GIPS-compliant performance track record), and a dollar-weighted return (to most accurately report to each client his/her true investor return). Fortunately, most performance reporting software tools are capable of doing at least some version of both, though not all are capable of each (and/or may use different methodologies to calculate TWR in particular, not all of which will necessarily be GIPS-compliant).

When considering a performance reporting tool, advisory firms should verify which options are available, and exactly how each system computes their performance numbers.

So what do you think? How do you calculate performance for clients?  Do clients generally understand the different ways fees can be calculated? Should performance be calculated in multiple ways depending on what the objective is? Please share your thoughts in the comments below!